Power Functions
Savanna is reading the trails of asteroids, comets, and different our bodies that fly thru space. She notices that as a positive comet receives toward the earth, the route of the comet curves and movements away. Savanna desires to create mathematical features of the comet and asteroid paths as they arrive toward the earth. This manner, she will expect the trails of positive comets and asteroids. Savanna can use her understanding of strength features to create equations primarily based totally at the paths of the comets.
A strength characteristic is with inside the shape of f(x) = kx^n, where k = all actual numbers and n = all actual numbers. You can extrade the manner the graph of a strength characteristic seems through converting the values of k and n.
If n is more than zero, then the characteristic is proportional to the nth strength of x. This essentially approach that the 2 graphs might appearance the same. Here is a graph showing x^four:
So on this graph, n is more than zero. Here is the graph of f(x) = x^four. There isn’t any distinction among the 2 graphs.
If n is much less than zero, then the characteristic is inversely proportional to the nth strength of x. That approach you’ll see the graph form of flipped. Let’s study our graph of x^four once more.
It is straightforward to confuse strength features with exponential features. Both have a fundamental shape this is given through parameters. Both bureaucracy appearance very similar. In exponential features, a set base is raised to a variable exponent. In strength features, however, a variable base is raised to a set exponent.
More details
The parameter a serves as a easy scaling factor, shifting the values of xb up or down as a will increase or decreases, respectively
The parameter b , known as both the exponent or the strength, determines the characteristic’s prices of boom or decay. Depending on whether or not it’s far advantageous or poor, an entire wide variety or a fraction, b may even decide the characteristic’s common form and conduct.
More so than different easy households like lines, exponentials, and logs, individuals of the strength own circle of relatives can show off many special behaviors.
Notice that whilst b = 0 the characteristic simplifies to f(x) = ax0 = a1 = a , a consistent characteristic with an output of a for each input. When b > 0 , f(0) = a0b = 0 . That is, each strength characteristic with a advantageous exponent passes thru (0, 0) . When b < 0> f(0) is undefined. (Remember, x–b = 1 / xb .) Thus, strength features with poor exponents don’t have any y-intercepts. Power features with poor, entire wide variety exponents like x–1 or x–2 are easy examples of rational features, and for those features x = 0 is an instance of a singularity.
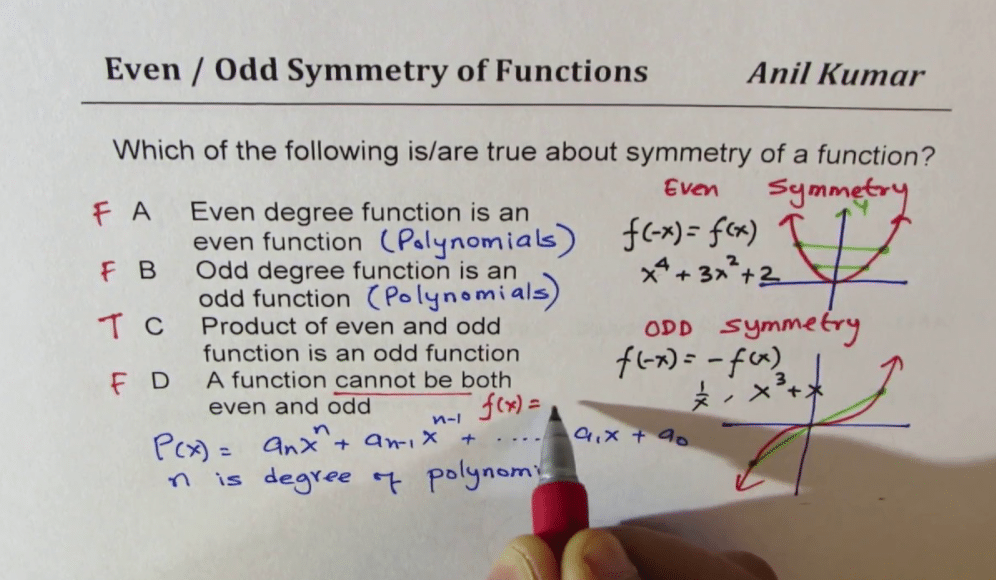
Even powers.
If b is a a fair entire wide variety like b = –2, four, 10, etc., then for any input x we can have f(–x) = a(–x)b = a(–1)b(x)b = a(x)b = f(x) , since –1 raised to a fair strength is 1 . The characteristic has a positive symmetry: Its outputs for any x are precisely similar to its outputs for –x . We name any characteristic with this conduct a fair characteristic, with even powers serving because the archetype.
Odd powers.
If b is a an bizarre entire wide variety like b = –1, 3, 7, etc., then for any input x we can have f(–x) = a(–x)b = a(–1)b(x)b = a(–1)(x)b = –f(x) , since –1 raised to an bizarre strength is –1 . The characteristic has a positive anti-symmetry: Its outputs for any x are precisely the alternative of its outputs for –x . We name any characteristic with this conduct an bizarre characteristic, with bizarre powers serving because the archetype.
The distinction among bizarre or even powers best suggestions on the variations amongst strength features.
Another beneficial difference separates features with entire wide variety (integer) powers from people with fractional powers. (We go away the attention of irrational powers to calculus.)
Integer powers.
We’ve already referred to the symmetry/anti-symmetry of even/bizarre integer powers. There is likewise a key distinction among advantageous and poor integer powers. We’ve referred to that each one advantageous powers by skip thru (0, 0), even as all poor powers have a singularity at x = 0 . When we prepare the even/bizarre opportunities with the advantageous/poor opportunities for integer powers, we discover 4 awesome instances for boom and decay.
Cases for integer powers:
Fractional powers.
It does not make any experience to differentiate among “even” and “bizarre” fractional powers – the ones phrases refer best to integers.
It does makes experience to speak approximately advantageous and poor fractional powers, however, and this difference is once more vital in figuring out common conduct.
Another difference – a brand new one – additionally comes into play whilst we remember fractional powers. Suppose that the fractional strength m/n has been decreased to lowest phrases (all not unusual place elements within side the numerator and the denominator were cancelled). To calculate xm/n, we continue in steps: 1) Find the n th root of x (x1/n = ); 2) Raise it to the m th strength. The 2d step is straightforward: We can boost any wide variety to an integer strength. The first step, however, is complex for poor x : We can’t meaningfully ask for a fair root of a poor wide variety. Thus, the allowable inputs (domains) of fractional powers depend upon whether or not n is even or bizarre.
Considering this in aggregate with the advantageous/poor opportunities, we yet again locate 4 awesome instances for boom and decay (every with numerous sub-instances).
How around taking into consideration these approaches as we introduce our children to the globe of mathematics:
1. Declare regarding mathematics – Our kids like to imitate us; if we send an adverse message to them about our very own experiences, they may approve it and believe their very own experiences will certainly coincide. So do not groan regarding just how much you hated math in college, and certainly do not make flippant remarks like you were fortunate you found out to count as that is all you need in life.
2. Treat mathematics as exciting – Usage bright challenge describes basic principles of enhancement and subtraction. This is the beautiful thing about mathematics. It opens you as much as a world of chances. I used my flooring ceramic tiles to discuss the concept of location. Can use apples as well as other colourful fruits to show addition as well as reduction easily. Portions are better recognized when they can be pictured, so utilize their everyday image puzzles to clarify fractions – they are pieces of a whole, so why not? The same point relates to items of apples and also oranges.
Blink cards and video games likewise spark interest – some teachers/parents advocate the math brilliant card games. Download several of the cost-free mathematics worksheets and also sources online; some are highly imaginative. I can not emphasize this adequate. The web has a wealth of enjoyable mathematics resources.




