Definitions
Before stepping into the definition of a horizontal asymptote, let’s first cross over what a feature is. A feature is an equation that tells you the way matters relate. Usually, features inform you how y is associated to x. Functions are regularly graphed to offer a visual.
A horizontal asymptote is a horizontal line that tells you the way the feature will behave on the very edges of a graph. A horizontal asymptote isn’t always sacred ground, however. The feature can contact or even move over the asymptote.
Horizontal asymptotes exist for features in which each the numerator and denominator are polynomials. These features are called rational expressions. Let’s examine one to peer what a horizontal asymptote appears like.
So, our feature is a fragment of polynomials. Our horizontal asymptote is y = zero. Look at how the feature’s graph receives nearer and in the direction of that line because it tactics the ends of the graph. We can plot a few factors to peer how the feature behaves on the very a long way ends.
Do you notice how the feature receives nearer and in the direction of the line y = zero on the very a long way edges? This is how a feature behaves round its horizontal asymptote if it has one. Not all rational expressions have horizontal asymptotes. Let’s communicate approximately the guidelines of horizontal asymptotes now to peer in what instances a horizontal asymptote will exist and the way it’ll behave.
Horizontal Asymptotes Rules
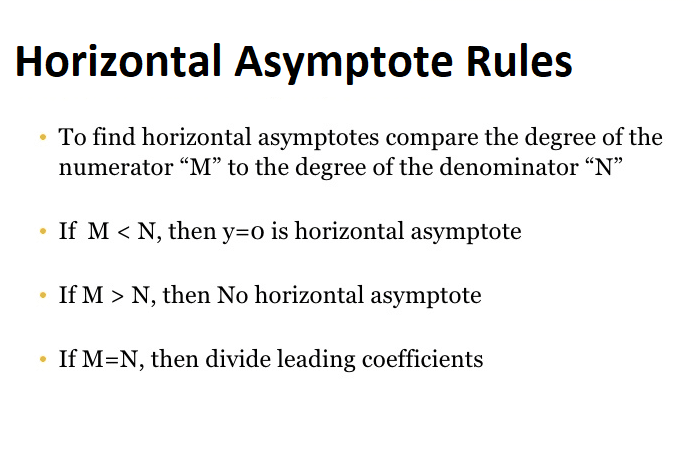
There are 3 guidelines that horizontal asymptotes comply with relying at the diploma of the polynomials concerned within side the rational expression.
Our feature has a polynomial of diploma n on pinnacle and a polynomial of diploma m at the bottom. Our horizontal asymptote guidelines are primarily based totally on those stages.
- When n is much less than m, the horizontal asymptote is y = zero or the x-axis.
- Also, when n is same to m, then the horizontal asymptote is same to y = a/b.
- When n is more than m, there may be no horizontal asymptote.
The stages of the polynomials within side the feature decide whether or not there may be a horizontal asymptote and in which it’ll be. Let’s see how we are able to use those guidelines to determine out horizontal asymptotes.
Whereas vertical asymptotes are sacred ground, horizontal asymptotes are simply beneficial suggestions.
Whereas you may by no means contact a vertical asymptote, you may (and regularly do) contact or even move horizontal asymptotes.
Two cents
Whereas vertical asymptotes suggest very particular behavior (at the graph), generally near the origin, horizontal asymptotes suggest popular behavior, generally a long way off to the perimeters of the graph.
In different words, horizontal asymptotes are distinctive from vertical asymptotes in a few pretty large ways.
Find the horizontal asymptote of the subsequent feature:
First, be aware that the denominator is a sum of squares, so it does not issue and has no actual zeroes. In different words, this rational feature has no vertical asymptotes. So we are k on that front. As referred to above, the horizontal asymptote of a feature (assuming it has one) tells me more or less in which the graph will being going whilst x receives truely, truly large. So I’ll examine a few very large values for x; that is, at a few values of x which can be very a long way from the origin.




